|
Chapter |
The
conservation of energy is one of the most basic physics laws, for the
electromagnetic field, the energy conservation equation is as follows:
(15.1)
In
which 
is the mass
density, 
is the field
velocity.
The mass density and
energy density have the following relationship:
In which u is the
electromagnetic field energy density.
From
equation (14.15) and (14.16), we have:
(15.2)
And
(15.3)
Thus:
(15.4)
And
(15.5)
Thus:
(15.6)
And
(15.7)
Combine (15.6) and
(15.7), thus:
(15.8)
Let us assume that
the electromagnetic energy is conserved, therefore, we have:
(15.9)
Thus:
(15.10)
Thus, we have:
(15.11)
As we know:
(15.12)
(15.13)
Let us define:
(15.14)
(15.15)
From the
electromagnetic field energy conservation equation, then we can get the
following equation:
(15.16)
Then we can rewrite
the electromagnetic field as follows:
(15.17)
(15.18)
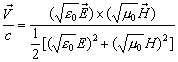(15.19)
(15.20)
(15.21)
(15.22)
|
What
is the electron spin?
ISBN 0-9743974-9-0 Copyright © 2003 Gengyun Li All rights reserved http://www.electronspin.org |